Our Car Audio Masterclass series offers a comprehensive introduction to the essential concepts of car audio systems, covering everything from basic terminology to advanced measurement techniques. To learn more, check out the other articles in the series:
- Car Audio Masterclass 101.1 – Audio Basics: Learn key audio terms and concepts.
- Car Audio Masterclass 101.2 – Understanding Basic Electricity: Explore voltage, current, and resistance.
- Car Audio Masterclass 101.3 – Electric Circuits: Understand series and parallel circuits.
- Car Audio Masterclass 101.4 – Magnetism: Discover the role of magnetism in speakers.
- Car Audio Masterclass 101.5 – Direct and Alternating Current: Compare DC and AC currents.
- Car Audio Masterclass 101.6 – AC Calculations: Dive into AC waveform calculations.
- Car Audio Masterclass 101.7 – The Decibel: Learn about decibel measurements.
- Car Audio Masterclass 101.9 – Time and Frequency Domain Measurement: Explore frequency and time measurements.
- Car Audio Masterclass 101.10 – Digital Multimeter: Learn to use a multimeter in car audio.
Our last lesson talked about decibels from the context of compressing large ratios into manageable numbers. In this article, we will expand on some basic concepts of loudness and then look at how adding more speakers and power to an audio system increases the output level.
Sound and Volume Levels
If a speaker is playing at a specific volume, it’s crucial to understand what’s required to increase the output. Science and simple math allow us to perform these calculations.
Let’s start with a typical 6.5-inch coaxial speaker. We’ll consider a speaker mounted in a car audio display board at a store. Providing this speaker with music at a power level of one watt might produce 88 dB SPL. Though we will get into speaker specification details later, this output level would be measured at a distance of 1 meter from the mounting surface, and rather than music, we’d be playing pink noise. It’s crucial to understand the details of the test conditions when quoting specifications and measurements.
If we decrease the power to 0.1 watts, the output will drop to 78 dB SPL. If we increase the power to 10 watts, the output should increase to 98 dB SPL. If we increase or decrease power by a factor of 10, output increases or decreases by 10 dB SPL. This relationship doesn’t extend infinitely, as there is a limit to how loud a speaker can play. We’ll cover that in a future lesson.
What happens when we double the power to a speaker? If we go from one to two watts, the output should increase by 3 dB SPL. The same applies the other way around: if we decrease the power from one to 0.5 watts, the output decreases by 3 dB SPL.

Power and Output Level Math
If we go back to the prior lesson on decibels, we’ll note that the ratio of doubling or halving a power number is equal to 3 dB. The formula to calculate a change in level based on a change in power is 10 x LOG (P1 / P2). If we go from 1 to 2 watts, we have a ratio of 2, which equals 3.01 dB. If we decrease power from 2 to 1 watt, the ratio is 0.5, which results in -3.01 dB.
It’s important to understand that this is a ratio we are comparing. Going from 10 to 20 watts is a doubling, so we get 3.01 dB. On the other hand, going from 20 to 30 watts only provides a ratio of 1.5, resulting in an increase of only 1.76 dB. That’s audible but pretty subtle.
An increase from 100 to 110 watts represents an increase of only 0.41 dB or a ratio of 1.1. Going from 1,000 to 1,010 watts is almost imperceptible at 0.04 dB. Car audio enthusiasts who worry about differences of a few dozen watts when comparing powerful amplifiers are wasting their time. For example, if one amp produced 2,500 watts and a second produced 2,250 watts, the difference is only 0.09 dB. You will never hear that with music. If you’re entering SPL competitions, then maybe that matters. It’s FAR more likely that amplifier efficiency is a more significant factor for our bass head friends.
Predicting Output Levels
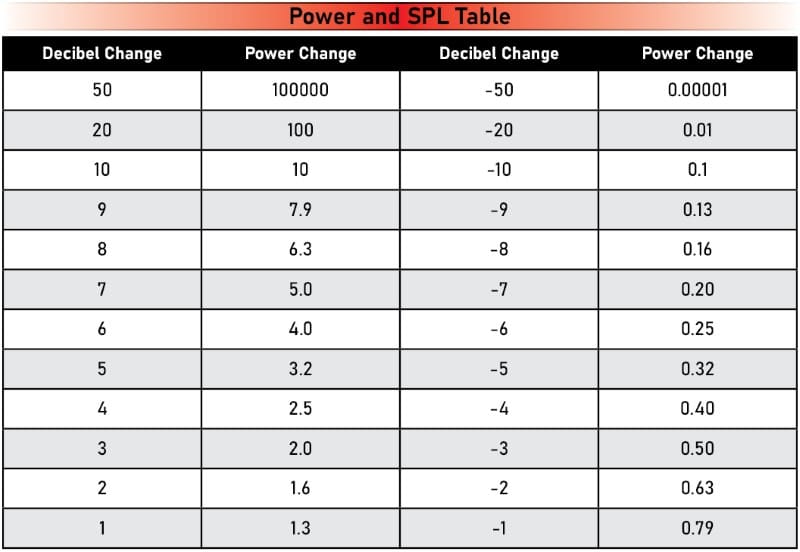
The table above shows the power multiplication factor required to increase or decrease a speaker’s output. We will reference our 88 dB SPL at 1 watt measured at 1 meter. If we want the speaker to play at 95 dB, which is 7 dB louder, then we need to increase the power by five times, which would be 5 watts.
You would be surprised by how loud 88 dB SPL is. Knowing that, if you have a radio playing at a 60 dB level, which would be quiet, your amplifier is likely producing well under a watt of power.
SPL Levels and Sound Perception
As we have investigated, human hearing functions logarithmically in terms of power and perception. Significant power increases are needed to create a perceptible difference in sound level.
Let’s define a few factors concerning human hearing. First, 1 dB SPL is considered the slightest change in volume that the average human can detect. If you want to test this, you will likely need a quiet environment. You will likely need to play a sound in the more sensitive range of human hearing. Frequencies between 2 and 5 kHz would work best. We’ve tried, and the difference, even at precisely 3.5 kHz, takes concentration to detect.
An increase or decrease of 3 dB SPL is considered a doubling or halving of sound intensity, but not in perceived volume level. Over the years, we’ve heard a 3 dB SPL change in volume level as being perceivable across most of the audible frequency range. However, an increase or decrease of 3 dB SPL is NOT perceived as twice or half as loud. Many audio enthusiasts confuse the 3 dB doubling or halving of power as a doubling or halving of how loud something is. Picking out a decrease by 3 dB is easier than picking out 1 dB.
A change in volume level of 10 dB SPL is considered to be twice or half as loud with respect to human hearing. However, the difference is somewhat subjective.
More Speakers, More Sound
The second consideration is the number of speakers in a system. If we have 1 watt of power driving one speaker and measure 88 dB of output, how loud will the system be with two speakers? If we add a second speaker beside the first and split that 1 watt of power between the two, we should measure 91 dB, which is an increase of 3 dB. If we provide each speaker with 1 watt of power, the output increases another 3 dB to 94 dB SPL.
To recap, if we add another speaker driven by the same amount of power, effectively doubling the power the system is using, the output level goes up by 6 dB SPL.
If we want another 6 dB of output, we must double the power and the cone area again. So, four speakers, each receiving 1 watt of power, would produce 100 dB SPL. We can use that chart above to determine how loud four speakers would be if they shared 1 watt of power. Look at the Power Change column for the number 4. Compared to our reference level, four times as much power increases output by 6 dB SPL. If we reduce the power by 0.25, the output level will decrease by 6 dB SPL.

How Loud is Loud?
Where you listen to music dramatically affects how much power it takes to achieve a certain volume level. For example, in 1976, The Who played a concert that was said to be 120 dB SPL. They needed 76,000 watts of power to achieve this. On the other hand, a single 13,000-watt car audio amplifier can produce sound pressure levels exceeding 180 dB SPL inside a vehicle designed specifically for car stereo SPL contests.
Out of curiosity, we can make a mathematical prediction about how loudly that system would play with only 1 watt of power to all its speakers. A ratio of 13,000:1 is 41.14 dB. So, theoretically, the system would play at 138.86 dB SPL. It’s likely a bit louder as speakers decrease efficiency at high power levels. A fun test would be to use a car radio rated to produce 20 watts of power into four speakers and see how loud one of these vehicles would play. The math says 160.97 dB SPL.

Power, Speakers and SPL Levels
Let’s recap a few key takeaways from this lesson. Doubling the power to a speaker results in an increase of 3 dB, correlating to an increase in output of 3 dB SPL. Halving the power to a speaker leads to a decrease of 3 dB, reducing the output by 3 dB SPL. Ten times as much power is a 10 dB increase, while one-tenth as much power is a 10 dB decrease.
Doubling the number of speakers in a system increases the output by 3 dB SPL. If those new speakers accompany a doubling of power, the total increase is 6 dB SPL.
A change in volume level of 1 dB SPL is difficult, but not impossible, to discern. A change in volume level of 3 dB SPL is audible across most of the audible frequency range. A change in sound pressure level of 10 dB is considered twice or half as loud.
The following Car Audio Masterclass lesson will discuss measuring audio signals in the time and frequency domains.