Our Car Audio Masterclass series offers a comprehensive introduction to the essential concepts of car audio systems, covering everything from basic terminology to advanced measurement techniques. To learn more, check out the other articles in the series:
- Car Audio Masterclass 101.1 – Audio Basics: Learn key audio terms and concepts.
- Car Audio Masterclass 101.3 – Electric Circuits: Understand series and parallel circuits.
- Car Audio Masterclass 101.4 – Magnetism: Discover the role of magnetism in speakers.
- Car Audio Masterclass 101.5 – Direct and Alternating Current: Compare DC and AC currents.
- Car Audio Masterclass 101.6 – AC Calculations: Dive into AC waveform calculations.
- Car Audio Masterclass 101.7 – The Decibel: Learn about decibel measurements.
- Car Audio Masterclass 101.8 – Speakers, Power, and SPL: Understand speaker power and sound levels.
- Car Audio Masterclass 101.9 – Time and Frequency Domain Measurement: Explore frequency and time measurements.
- Car Audio Masterclass 101.10 – Digital Multimeter: Learn to use a multimeter in car audio.
Our Car Audio Masterclass 101 series continues with a lesson on understanding the basic concepts of electricity. This knowledge is not just theoretical—it powers the radios, speakers, amplifiers and signal processors we all love. Let’s start with some simple electron theory and then move on to the terminology to help us confidently discuss car audio products.
What Is Electricity?
Even though it’s typically invisible, electricity is not a concept confined to textbooks. It’s present all around us: in our homes, cars and the devices we use every day. In the context of car audio, it’s a crucial element that powers the electronics and makes speakers work.
Almost all the matter in the universe is made up of atoms. Atoms are inconceivably small, at 10^-10 meters in size, yet they can combine to make solids, liquids and even gases. The space between atoms is critical to determining the physical characteristics of the matter. When packed together tightly, we get solids. When spread far apart, we get gases.
Atoms are made of three subatomic particles: protons, neutrons and electrons. The number of protons in an atom’s nucleus (center) is the defining factor in determining what element the atoms have combined to create. For example, an oxygen atom has eight protons, and a nitrogen atom has seven. Usually a similar number of electrons are orbiting the nucleus. However, as we are about to learn, these negatively charged electrons like to hop from atom to atom.

Electricity and Electron Theory
At the most fundamental level, the presence or lack of electrons in an atom’s outer layer determines its charge. This simple yet profound fact is the basis of all electrical phenomena. Electrons have a negative charge, and protons in the nucleus of the atom have a positive charge. When an electron leaves its orbit around the nucleus, the atom is said to have a positive charge. This is because there are more positive protons than negative electrons left.
Electricity is the movement of electrons from one atom to another. Why do electrons move? When we have a power source like a battery, there is an overabundance of electrons in the atoms connected to the negative terminal and an electron deficiency at the positive terminal atoms.
What are Conductors?
A conductor is a material that allows electrons to move from atom to atom easily. Materials like copper, silver, platinum and gold are excellent conductors because they typically have only a single electron in the outer layer of each atom. This isolation makes it easy for that electron to jump to the next atom and for a new electron from an adjacent atom to fill the missing location.
Voltage
The first term we’ll discuss is voltage. In simple terms, this describes an imbalance of electrons. If millions of extra electrons are at the negative terminal of a battery, then we would measure a very small voltage across the battery terminals. We measure a larger voltage if quadrillions of electrons are present at the negative terminal.
Voltage is akin to electrical pressure or potential energy. More voltage means more electrons are piled up and eager to return to the positive terminal. The electrons move faster and more vigorously when the imbalance is more significant.
To be clear, the actual size of the electron imbalance in a battery depends on factors other than voltage. For example, a hearing aid battery won’t store as much energy as a car battery. A car battery pales in energy storage capacity compared with the battery that powers an electric vehicle. Electric vehicle batteries are small compared with energy storage facilities at the Edwards & Sanborn Solar Plus Storage Project and the Moss Landing Energy Storage Facility.

Voltage is described using the SI unit volt. We use an uppercase V after the number to quantify the potential between two points, e.g., 12 V.
Voltage is always measured between two points in a circuit or device. If we put the leads of a voltmeter on the same connection, we shouldn’t measure any significant voltage difference. If we move a test lead to the other side of a resistor, transistor or diode, and current is flowing through that device, we should measure a voltage difference.

Current
Current quantifies the rate of electron flow through a conductor. The SI unit for current is the ampere, or amp. One amp is equivalent to the movement of 6.242 x 10^18 electrons per second through a conductor. We use an uppercase ‘A’ to denote the flow of electrons in amps. Interestingly, in calculations, current is represented by the uppercase letter ‘I,’ originating from the French term ‘intensité de courant,’ meaning intensity of current.
Understanding the rate of electron flow is crucial for describing how much work is being done in an electric circuit. A battery sitting on a table isn’t performing any significant work. However, work occurs when there is a path for electrons to flow. For instance, a light bulb connected to a battery will light up when electrons flow through its filament. Similarly, an LED emits light when connected to a power source like a battery.”

Like voltage, current is measured between two points in a circuit. However, we must make provisions for the current to pass through the measuring device. An ammeter has a very low internal resistance, often a hundredth of a thousandth of an ohm. If we put the leads of an ammeter directly across a power source, it will act as a short circuit and blow its internal fuse or damage the supply circuit. Ammeters must be wired in line with the load. We’ll get into this again later on.
Resistance
The third term we must understand is resistance. Resistance represents the opposition to the flow of electrons through a conductor or medium. Resistance values are described using the SI unit ohm. We use the Greek letter omega (Ω), which looks like a horseshoe after the number. When considering resistance in equations, we use a capital R.
At the atomic level, high-resistance materials make it hard for electrons to jump from one atom to another. Silver is the most conductive metal on Earth, followed by gold and copper. Gold is often considered to be an ideal conductor. The main reason gold is used is its inertness and corrosion resistance.
At the upper end of the resistance scale, we move away from objects being conductors to them being insulators. Insulators are materials that don’t allow current to flow easily. Some better insulating materials are rubber, glass, oil and air. These materials can be in direct contact with a conductor yet won’t allow electrons to move through them easily. Typically, we wrap conductors like copper in insulators like rubber or PVC.

An ohmmeter can measure the resistance of almost any component. However, we can’t always make a measurement when a device is in a circuit or current is passing through it. Typical ohmmeters are very bad at measuring resistance values below about 2 ohms. The circuitry they use to measure is not very accurate below this range. If you short the leads of an ohmmeter together, you should get 0 ohm. However, we usually see about 1 ohm displayed on the screen.
The Water and Bucket Analogy
Though we’ve mentioned it in other articles, we want to ensure that the Car Audio Masterclass series is complete. As such, we will reintroduce the theory of using a bucket of water and a hose to describe electricity.
Imagine a bucket sitting on top of a stepladder. The bucket is full of water, which represents our negative electrons. The size of the bucket represents the capacity of our battery. A small bucket, like you’d see in a fairy tale wishing well, doesn’t store much water. A tanker truck, on the other hand, might be able to fill a swimming pool.

Voltage, Current and Resistance
A hose, which is our conductor, is attached to the bottom of the bucket. The size of the hose determines how quickly we can empty the bucket or water tank. A tiny hose the diameter of a drinking straw won’t drain a tanker truck very quickly. Conversely, a 2-inch fire hose will. In this analogy, the fire hose has less resistance.
The volume of water flowing through the hose each second is similar to describing current or amperage. A larger hose allows more water to flow, and a conductor with lower resistance allows more electrons to flow.

If we added a water wheel to the hose outlet, that would represent our load. The faster we can deliver water to the wheel, the more work it does.
If we increase the size of the bucket, more pressure will be created at the base of the bucket, pushing more water through the hose. This increase in water flow would make the water wheel spin faster.

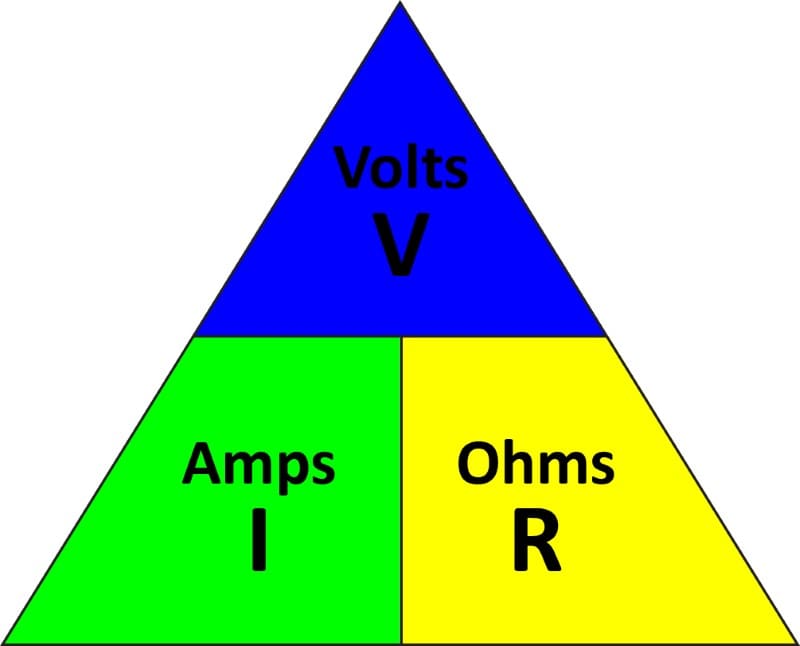
Ohm’s Law
Let’s combine the concepts of voltage, current flow, and resistance to explain Ohm’s law. This law describes the fixed relationship between voltage, current, and resistance in a circuit, which is crucial for understanding all electric circuits.
Ohm’s law states that if there is a potential of 1 volt across a resistance of 1 ohm, 1 amp of current will flow through that resistance. Generally, the voltage across a resistance is proportional to the current flowing through the resistance and inversely proportional to the resistance value.
We can look at this another way: If we apply 1 volt to a load with a resistance of 1 ohm, 1 amp of current will flow. This is the most common explanation of Ohm’s law.
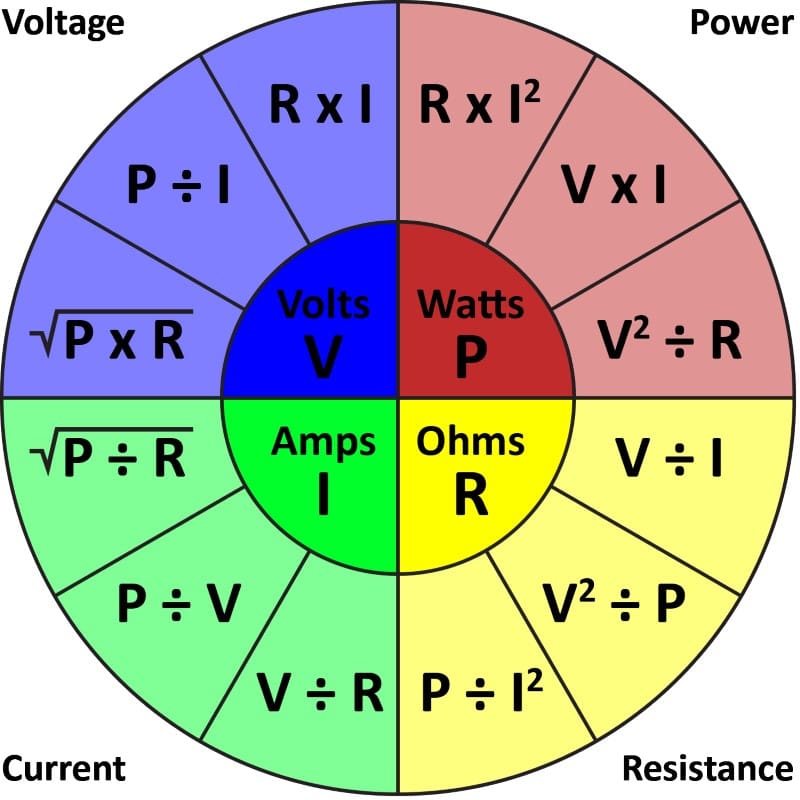
We can extract three equations from Ohm’s law. The first calculates voltage based on resistance and current flow: V = I x R. The second formula calculates current flow for given voltage and resistance measurements: I = V ÷ R. Finally, we can calculate a resistance value if we know the voltage across the resistor and the current flowing through the resistor: R = V ÷ I. Knowing these equations by heart is crucial to understanding basic electrical theory.
Work and Power
In electrical terms, power is the measure of work. Work can be mechanical, such as producing motion, or it can produce heat or light.
The SI unit of power is the watt, represented with a capital W. Specifically, a watt defines the rate at which work is done or energy is transferred. One watt is equivalent to 1 joule of energy consumed in one second. A joule is equivalent to the heat produced when 1 amp of current flows through a 1-ohm resistor in one second.
Suppose we have a battery connected to a light bulb. In that case, electrons flow from the battery’s negative terminal through the conductors, through the bulb’s filament to the positive terminal of the battery.
There are many equations related to calculating power in an electrical circuit. In all cases, the time base for these calculations is one second. The first equation is P = I x V. Looping back a few sections, we know that 1 volt of electricity will be present across a 1-ohm resistor when 1 amp of electrical current flows through the resistor. Our new formula tells us that 1 watt of power is consumed when 1 amp of current flows through a load or device with 1 volt across it. A car battery measuring 12 volts and delivering 200 amps of current to start your vehicle provides 2,400 watts of power: 12 V x 200 A = 2,400 W.
Power Calcuation Equations
The second power formula we should know is P = V^2 ÷ R. If we measure 6 volts across a resistor with a value of 10 ohms, we can calculate that that resistor is dissipating 3.6 watts of power. The math is (6 V x 6 V) ÷ 10 Ω, or 36 ÷ 10, which is 3.6.
Finally, we can calculate power if we know current and resistance using P = I^2 x R. Five amps of current flowing through a 10-ohm resistor produces 250 watts of heat. The math is (5 A x 5 A) x 10 Ω = 250 W.
We’ve created a tool to help you understand all the equations related to making calculations relevant to car audio systems.
Basic Electrical Theory
After gaining a solid understanding of the basic terminology of volts, amps, ohms, and watts, you can discuss the intricacies of automotive audio and electrical systems more intelligently. Soon, we will revisit these terms and apply them directly to explain how amplifiers and speakers function.
The next chapter of the Car Audio Masterclass will deal with electric circuits. See you there!

